3D Reslicing using COMKAT image tool (basic)

From COMKAT wiki
Revision as of 21:26, 10 August 2012 by DylanSu (talk | contribs)
Jump to navigation Jump to search

Reslicing 3D image volume using COMKAT image tool (basic)

Overview

Reslicing a 3D (or 3D vs time) image dataset can be accomplished using various components of COMKAT including the function sliceVolume() which is a method of ImageVolumeData. This example explains how to create a 2D image by slicing from a volume at a position, plane orientation, and magnification specified by the user. The approach is to load the image volume dataset into an instance of an ImageVolumeData (abbreviated IVD) object and to use the sliceVolume() method.

Background

sliceVolume() is a mex-file written in c with an interface to MATLAB that makes the operation particularly efficient. COMKATImageTool uses sliceVolume() and you can use it too.

Approach I. Demonstrate the method for coordinate transformations

Create an instance of an IVD and load it with an image volume. 

  ivd = ImageVolumeData(); % create an instance of an ImageVolumeData object
  ivd = read_DICOM(ivd, pathName, fileName); % load volume into an instance of IVD object;


Create lists of indices of all pixels in the 2D slice (rectangular grid) that we are creating 

  [i, j] = meshgrid(0 : Nc-1, 0 : Nr-1); % i and j will be 2D arrays, meshgrid is a function built into MATLAB
  ij = [c(:)’ ; r(:)’];  % make matrix, each column corresponding to a single pixel in the slice we are creating

The first and second rows of ij are the indices corresponding to column and row indices of all voxels in the desired slice. So the dimension of ij is 3 x ( # of desired voxels ).

For example, the first column of ij could be [ 0 ; 0 ] ; the second column could be [ 0 ; 1 ] , etc.


Compute the physical x,y,z locations, in mm, from pixel indices according to the DICOM coordinate system ref [1] p. 275.

This is a two-step process. The first step is to compute the coordinate transformation matrix. Note that pixel spacing/zoom, orientation, and position for the slice are specified in the transformation matrix. 

  M = ( Insert the method for generating the transformation matrix );


The second step is to use the transformation matrix to calculate the physical (mm) location of each pixel in the desired slice 

  xyz = M * ij;   % xyz is a matrix consisted of three rows the physical x, y, and z location (m) of all the desired voxels. The dimension of xyz is 3 x ( # of desired voxels ).


These xyz locations are the same as those in the image volume that is being sliced to make the 2D image. From these xyz locations, we find the corresponding 3D indices, (u,v,w), into the volume. This uses the transformation matrix for the volume, Mhat, that relates the indices to the xyz physical location. This is analogous to M used for the desired slice but here the pixel spacing, orientation, and position indicate how the volume data are stored.

Specify the matrix for reverse mapping ( index space of the original image volume (uvw) --> xyz ) 

  Mhat = ( Insert the method for generate the mapping);


Calculate voxel indcies into the volume corresponding to xyz physical location 

  uvw =  inv( Mhat ) * xyz;    % uvw is the matrices consisted of the indices in the domain of the original volume

uvw is a matrix consisted of three rows corresponding to row, column, and plane indices of the original volume data. The dimension of uvw is identical of that of xyz.


Separate uvw into the components 

    u = ( row indices in the domain of original of volume data );
    v = ( column indices in the domain of original of volume data );
   w = ( plane indices in the domain of original of volume data );

Therefore, the u, v, w are the first, second, and third rows of uvw.


Use sliceVolume() to interpolate the slice 

  slice = sliceVolume(idv, v, u, w, backgroundPixelValue, 'linear');

backgroundPixelValue is a value that used for the interpolation when it is nothing there for calculating the interpolation value. The value is a unscaled raw background value of images.

In gerneral, backgroundPixelValue = (scaled_pixel_value - rescale_intercept) / (rescale_slope) ;

e.g. For PET, the scaled background value is usually zero. 

==>    backgroundPixelValue  = ( 0 - rescale_intercept) / rescale_slope ;

So backgroundPixelValue can be calculated as follows: 

  s = get( ivd, 'VolumeFrameBufferScaleFactor');           % rescale_slope
  o = get( ivd, 'VolumeFrameBufferRescaleIntercept');  % rescale_intercept
  backgroundPixelValue  = - o / s;


Display new slice 

  figure, imagesc(slice); axis image % isotropic


Approach II. Use coordinateGen() to do the coordinate transformation

  • This should create same result as approach I but require fewer lines of coding since coordinateGen() does most things that are needed.


Read the image volume into an ImageVolumeData object 

  ivd = ( Insert the method for reading data );


Use coordinateGen() to generate uvw 

  [u, v, w] = coordinateGen(ivd, Nc, Nr, pixelSpacing, planePos, orientation); % Input the desired pixelSpacing, planePos and orientation matrices


Use sliceVolume() to interpolate 

  slice = sliceVolume(idv, v, u, w, backgroundPixelValue, 'linear');


Display slice 

  figure, imagesc(slice); axis image % isotropic


Example

ivd = (read data into IVD);

Nc = 370; Nr = 370;

pixelSpacingOrg = [1.5501, 1.5501, 1.5501];

planePosOrg = [-0.7751; -0.7751; 88.0000];

orientationInput = [1, 0, 0; 0, 1, 0; 0, 0, 1];


s = get(ivd, 'VolumeFrameBufferScaleFactor'); o = get(ivd, 'VolumeFrameBufferRescaleIntercept');

rawBackgroundPixelValue = -o/s;


[u, v, w] = coordinateGen(ivd, Nc, Nr, pixelSpacingOrg, planePosOrg, orientationInput); slice = sliceVolume(ivd, v , u , w, rawBackgroundPixelValue,'linear');

figure, imagesc(slice), axis image, colormap(hot) % show org image


% zoom zoomFactor = 3;

pixelSpacing = pixelSpacingOrg / zoomFactor; zoomShift = (370*1.5501)/2 * (zoomFactor-1)/zoomFactor; planePos = planePosOrg + [zoomShift; zoomShift; 0]; % org+zoom after zoom you should trans center or you see nothing

[u, v, w] = coordinateGen(ivd, Nc, Nr, pixelSpacing, planePos, orientationInput); slice = sliceVolume(ivd, v , u , w, rawBackgroundPixelValue,'linear');

figure, imagesc(slice), axis image, colormap(hot) % show org+zoom


% trans transMat = [-35.0; -5; 0]; planePosTrans = planePos + transMat; [u, v, w] = coordinateGen(ivd, Nc, Nr, pixelSpacing, planePosTrans, orientationInput); slice = sliceVolume(ivd, v , u , w, rawBackgroundPixelValue,'linear');

figure, imagesc(slice), axis image, colormap(hot) % show org+zoom+trans

% rot rotAngle = 25/180*pi; orientationInputRot = [cos(rotAngle), -sin(rotAngle), 0; sin(rotAngle), cos(rotAngle), 0; 0, 0, 1];

transMat2 = [370*0.5167/2; 370*0.5167/2; 0] - orientationInputRot*[370*0.5167/2; 370*0.5167/2; 0]; planePosTrans2 = planePosTrans + transMat2;


[u, v, w] = coordinateGen(ivd, Nc, Nr, pixelSpacing, planePosTrans2, orientationInputRot); slice = sliceVolume(ivd, v , u , w, rawBackgroundPixelValue,'linear');

figure, imagesc(slice), axis image, colormap(hot) % show org+zoom+trnas+rot


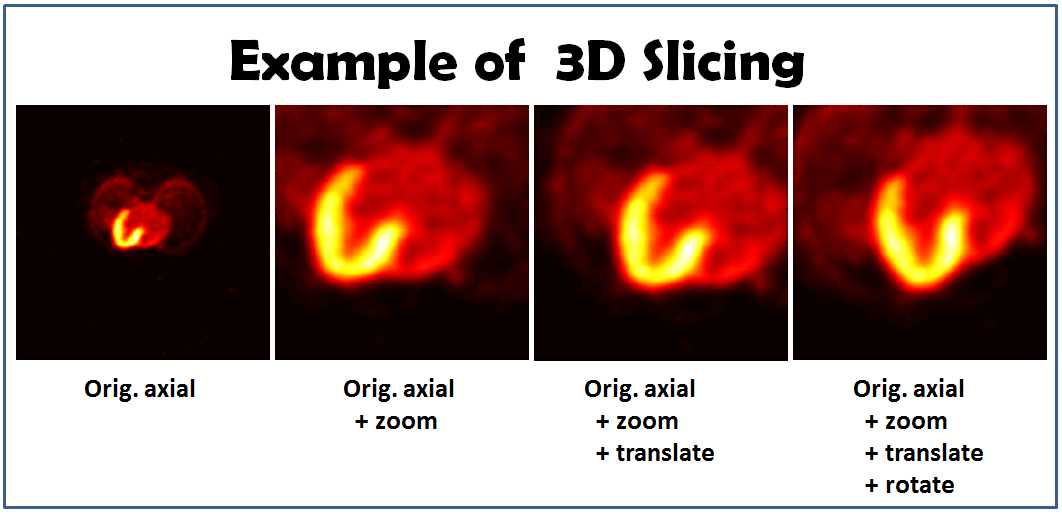
The slicing images may look like this:

Fig eg3Dslicing.png

Retrieved from "https://comkat.cwru.edu/index.php?title=3D_Reslicing_using_COMKAT_image_tool_(basic)&oldid=2200"